Readers who may have been faced with this question, let's answer it together and hopefully you can understand.
Here's the question...
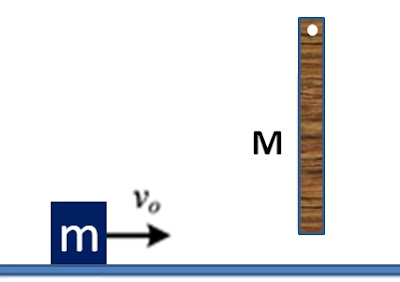
A beam (mass m) moves with an initial speed on a slippery floor. A homogeneous rod of mass M (M is much larger than m) and length L is hanging freely from the ceiling and is initially at rest (see figure). Bar M is pounded by block M.
Right after the impact, the rod swings and the block stands still.
- Check whether the above case is an elastic or inelastic collision.
- Determine the maximum height of a swinging homogeneous rod, if m = 2kg, M = 20kg and $v_o = 10$m/s
Momentum Solution:
The law of conservation of angular momentum, the angular momentum (angular momentum) before and after the collision must be the same. After the collision, the block is stationary while the rod rotates with an angular velocity .
See the picture
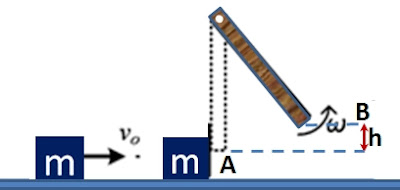
After being hit by the block, the rod rotates with an axis of rotation at one end, then the moment of inertia of the rod: $\frac13ML^2$.
From the conservation of angular momentum:$\begin{align*} L_{blk}+L_{btg}&=L'_{blk}+L'_{btg}\\mv_or+0&=0+I\omega \\mv_oL+0&=0+\frac{1}{3}ML^2\omega \\\omega&=\frac{3mv_o}{ML}\end{align*}$
Energi Kinetik:
Before the collision, only the block has kinetic energy. $\begin{align*}E_k=\frac12mv_o^2\end{align*}$
After Collision:
$\begin{align*}E'_k&=\frac12I\omega^2\\&=\frac12\left (\frac{1}{3}ML^2 \right )\left ( \frac{3mv_o}{ML} \right )^2\\&=\frac12\left (\frac{1}{3}ML^2 \right )\left ( \frac{9m^2v^2_o}{M^2L^2} \right )\\&=\left ( \frac{3m}{M} \right )\frac12mv_o^2\\&=\left (\frac{3m}{M} \right )E_{ko}\end{align*}$
Because M is much larger than m, the value of $\frac{3m}{M}$ < 1, then $E'_k < E_k$, meaning that there is energy lost, so the collision is inelastic.
Swinging rod height:
$\begin{align*}E_A&=E_B\\E_{kA}+E_{pA}&=E_{kB}+E_{pB}\\\left ( \frac{3m}{M} \right )\frac12mv_o ^2+0&=0+Mgh \\h=\left ( \frac{3m}{gM^2} \right )&\frac12mv_o^2\\h= \frac{3m^2v_o^2}{2gM^2 }&\textrm{ meters}\end{align*}$
We enter the numbers, m = 2kg, M = 20kg and $v_o $= 10m/s.
$\begin{align*}h&= \frac{3m^2v_o^2}{2gM^2}\\&= \frac{3(2)^2(10)^2}{2(10)(20) ^2}\\&= \frac{3(400)}{20(400)}\\&=0.15\textrm{ meters}\\&=15\textrm{ cm}\end{align*}$
The law of conservation of angular momentum, the angular momentum (angular momentum) before and after the collision must be the same. After the collision, the block is stationary while the rod rotates with an angular velocity .
See the picture
After being hit by the block, the rod rotates with an axis of rotation at one end, then the moment of inertia of the rod: $\frac13ML^2$.
From the conservation of angular momentum:$\begin{align*} L_{blk}+L_{btg}&=L'_{blk}+L'_{btg}\\mv_or+0&=0+I\omega \\mv_oL+0&=0+\frac{1}{3}ML^2\omega \\\omega&=\frac{3mv_o}{ML}\end{align*}$
Energi Kinetik:
Before the collision, only the block has kinetic energy. $\begin{align*}E_k=\frac12mv_o^2\end{align*}$
After Collision:
$\begin{align*}E'_k&=\frac12I\omega^2\\&=\frac12\left (\frac{1}{3}ML^2 \right )\left ( \frac{3mv_o}{ML} \right )^2\\&=\frac12\left (\frac{1}{3}ML^2 \right )\left ( \frac{9m^2v^2_o}{M^2L^2} \right )\\&=\left ( \frac{3m}{M} \right )\frac12mv_o^2\\&=\left (\frac{3m}{M} \right )E_{ko}\end{align*}$
Because M is much larger than m, the value of $\frac{3m}{M}$ < 1, then $E'_k < E_k$, meaning that there is energy lost, so the collision is inelastic.
Swinging rod height:
$\begin{align*}E_A&=E_B\\E_{kA}+E_{pA}&=E_{kB}+E_{pB}\\\left ( \frac{3m}{M} \right )\frac12mv_o ^2+0&=0+Mgh \\h=\left ( \frac{3m}{gM^2} \right )&\frac12mv_o^2\\h= \frac{3m^2v_o^2}{2gM^2 }&\textrm{ meters}\end{align*}$
We enter the numbers, m = 2kg, M = 20kg and $v_o $= 10m/s.
$\begin{align*}h&= \frac{3m^2v_o^2}{2gM^2}\\&= \frac{3(2)^2(10)^2}{2(10)(20) ^2}\\&= \frac{3(400)}{20(400)}\\&=0.15\textrm{ meters}\\&=15\textrm{ cm}\end{align*}$
